The Probability of Kunhuta

If you've lived so far under the sweet illusion that you possess what's known as common sense, allow me to correct that misunderstanding. This time, I'm set on crushing your mathematical intuition, stomping it into the ground, scraping it up with a knife, and triumphantly flushing it down a dry toilet!

You're in for a tricky puzzle, one that might very well have you catching yourself banging your head against the wall (yes, with your own head!) and digging your nails into places where hair used to shine just moments before. It's possible you'll tear up your college degree, burn the pieces, and smear the ashes across your face in a fit of despair. Be prepared for this and hide away any lighters and even walls from yourself. Don't end up claiming you hadn't anticipated this.

Puzzle

Let's dive in:

Task: There's a family. They have two children. One of them is a daughter.

Question: What's the probability that the family has two daughters?

Assuming that the chances of having a daughter or a son are equal, take a moment to think before you hastily answer, because I'm telling you now, “50%” is not correct. Don't read ahead and spoil the fun for yourself!

Puzzle with a twist

If you've solved the previous task, congratulations, but I have a new one for you, similar but with a slight variation.

Task: There's a family. They have two children. One of them is a daughter named Kunhuta.

Question: What's the probability that the family has two daughters?

I'll let you know that the answer to this puzzle is quite different from the first one. No joke! Think hard, ponder, and strain your brain. The correct answer will be revealed at the end of the article.

Solution

First, the puzzle without the twist. Given that sons and daughters are equally likely, the chance of the first child being a girl or a boy is 1:1. The same goes for the second child, resulting in four equally likely scenarios, each with a 25% probability:

• Girl + Girl
• Girl + Boy
• Boy + Girl
• Boy + Boy

A graphical representation can help, where each scenario occupies a corresponding 25% of the area:

Graphical illustration showing the four scenarios, with a black line surrounding the part of the diagram indicating the family has at least one daughter, excluding the Boy-Boy scenario. A pink square within this area represents the Girl-Girl scenario, making up one-third of the relevant area, indicating a 1 in 3 probability of the family having two daughters.

And now for the twist. Surprisingly, the probability significantly increases!

How is this possible? Another diagram can help explain. If every woman was named Kunhuta, the graph wouldn't differ from the previous one, and the solution would still be 1:3. However, women have various names. Suppose, for instance, that every sixth woman is named Kunhuta.

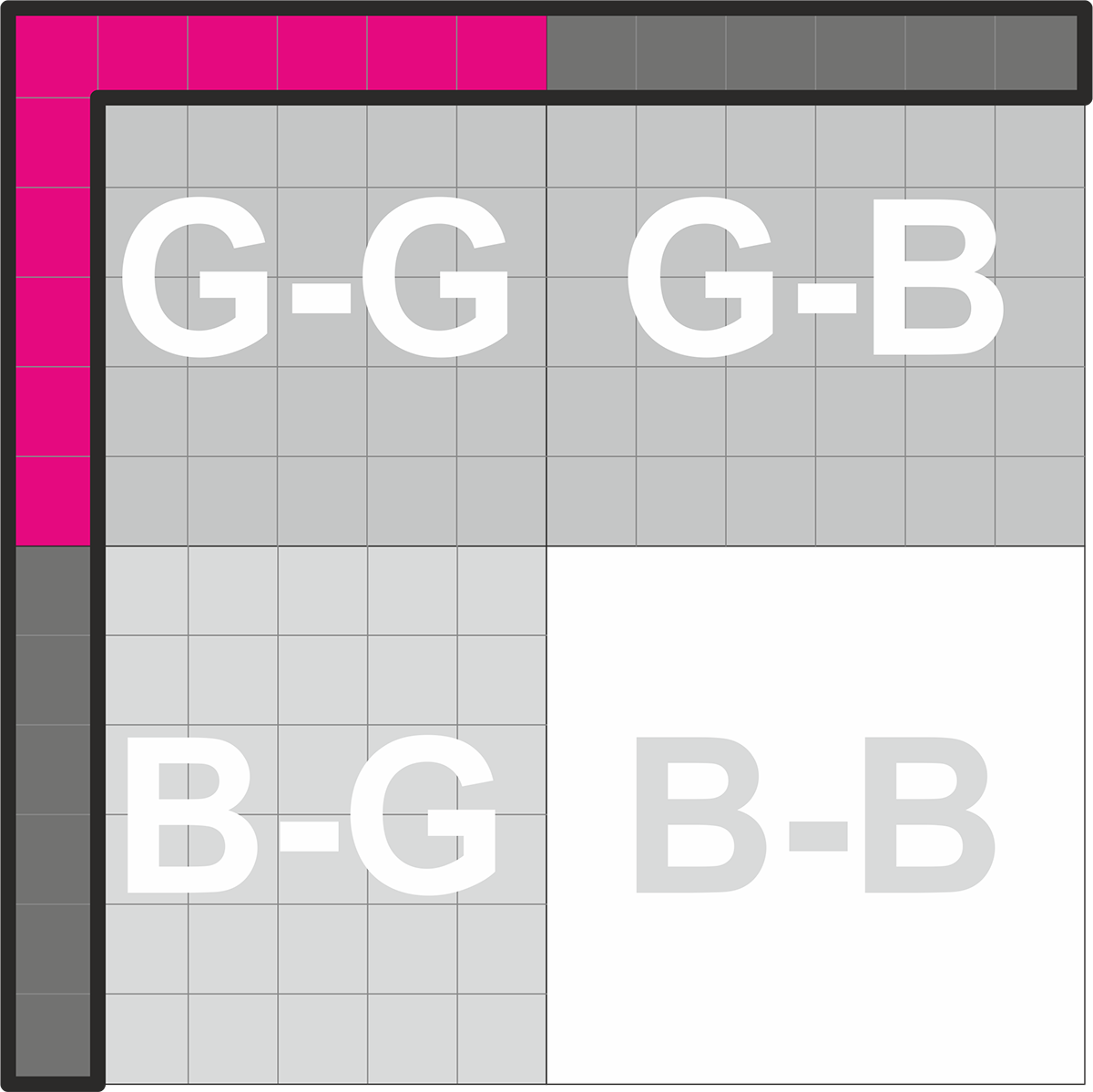
Graphical illustration showing the split of the Girl-Boy and Boy-Girl scenarios into sixths, with a darker area indicating a woman named Kunhuta and a lighter area indicating otherwise. The Girl-Girl square represents pairs of women, and since each has a 1 in 6 chance of being named Kunhuta, it's divided into sixths and then those remaining parts into sixths again. The chance that at least one of them is Kunhuta then becomes 11:36 (the pink area, made up of 11 mini squares out of 36).

The diagram shows that the pink area makes up about half of the entire outlined area. As the frequency of the name Kunhuta decreases in the population, the marked area will shrink, and the ratio will increasingly (and asymptotically) approach 1:2. According to the Czech Statistical Office, there are 4 Kunhutas in the country, making the answer to the question 49.99999076%.

The task doesn't clarify whether both daughters can have the same name (which is possible for half-siblings). Excluding this possibility would change the result insignificantly. I intentionally avoid presenting any equations, as I find it more beneficial to solve the puzzle graphically and intuitively. You can derive the equations as an exercise.